Fermat 2000 Q17

---

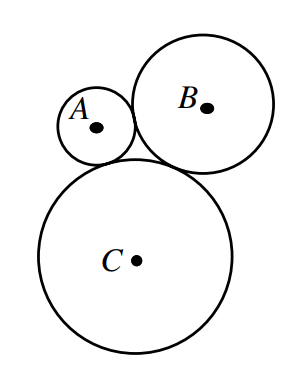
Problem Statement

Three circles have centres A, B and C with radii 2, 4 and 6 respectively. The circles are tangent to each other as shown. $\triangle ABC$ has

(A) $\angle A$ obtuse (B) $\angle B = 90^{\circ}$ (C) $\angle A = 90^{\circ}$ (D) all angles acute (E) $\angle A = \angle B$

Problem Link

Solution

$$AB = 2 + 4 = 6$$ $$AC = 2 + 6 = 8$$ $$BC = 4 + 6 = 10$$ $$BC^2 = AC^2 + BC^2 \text{ if and only if } \angle BAC = 90^{\circ}$$ $$\therefore \angle A = 90^{\circ}$$